L. G. Kreidik (translation from Russian T. S. Kortneva and G. P. Shpenkov)

3.1. Algebra of judgements

Let the oppositus and the semi-oppositus be equal on the level of relations and measures;

, . (1.33)

The relation between the elements and is composed by two sublevels-relations, namely, the relation between the bases and the relation between the superstructures. Experience of additive relations and operations states the additive algebra of basis-superstructure judgements.

Elementary additive algebra of the basis has the form

,

-------, (1.34)

,

where , if , and , if .

If one of the addends is a general judgement, then the sign "+" expresses an impracticable operation and we can only speak about tautology

,

-------- , (1.35)

.

Additive algebra of the superstructure is expressed by algebra of signs

,

-------, (1.36)

,

where , if a positive judgement is prevalent, otherwise, . If one of the addends is a neutral judgement without any sign, the tautology holds

,

--------------------------------(1.37)

.

Elementary additive algebra of the basis-superstructure is the first level of the spectrum of relations and operations of the basis-superstructure. On this level, the difference in the algebras of the basis and superstructure is insignificant and the algebras of affirmation and negation judgements are the same. The both algebras joined together determine the additive algebra of the basis-superstructure.

Now we will proceed to a description of multiplicative algebra of the basis. It states:

a) affirmation of some affirmation is affirmation

, (1.38)

b) affirmation of some negation is negation

, (1.39)

c) negation of some affirmation is negation

, (1.40)

d) negation of some negation is affirmation

. (1.41)

Multiplicative algebra of the superstructure is represented by two different envelopes of affirmation and negation.

Let the basis be affirmation. Positive affirmation of the envelope is expressed by the plus sign, negative affirmation of the envelope, by the minus sign. Plus and minus are the logical adjectives of a logical noun expressed by the basis. In the envelope of the affirmation basis

a) positive affirmation of the plus sign is the plus sign

, (1.42)

b) positive affirmation of the minus sign is the minus sign

, (1.43)

c) negative affirmation of the plus sign is the minus sign

, (1.44)

d) negative affirmation of the minus sign is the plus sign

. (1.45)

Combining the basis with the superstructure, we obtain the multiplicative algebra of the affirmation basis-superstructure

,

------ , (1.46)

.

Let us consider the multiplicative algebra of the negation superstructure.

Then, the basis is negation. Positive negation of the envelope is expressed by the plus sign and negative negation of the envelope, by the minus sign. Plus and minus signs in the negation superstructure differ from the corresponding signs in the affirmation superstructure, since they are signs of the negation basis. Algebra of the signs of the envelope states here that

a) positive negation of the plus sign is the minus sign

, (1.47))

b) positive negation of the minus sign is the plus sign

, (1.48)

c) negative negation of the plus sign is the plus sign

, (1.49)

d) negative negation of the minus sign is the minus sign

. (1.50)

Thus, the algebra of the signs in the multiplicative negation superstructure is negation of the multiplicative algebra of the affirmation superstructure:

----------,

----------,

------------------Þ---------------- Þ------ ---------(1.51)

----------,

----------.

Combination of the basis and superstructure gives the multiplicative algebra of the basis-superstructure of negation:

,

------ , (1.52)

.

Finally, we will discuss mixed multiplicative algebra of the affirmation-negation and negation-affirmation basis-superstructure. Let negation dominate on the basis level. On the superstructure level for complete contradiction, affirmation algebra will be assumed to be a dominant:

,

------- , (1.53)

,

.

Going to unit affirmations and negations, we obtain qualitative-quantitative multiplicative algebra of the basis-superstructure of:

a) affirmation

------- , , (1.54)

, ,

b) negation

------- , , (1.55)

, ,

c) affirmation of negation and negation of affirmation

,

------- , (1.56)

,

.

Elementary affirmation measures or briefly a, and elementary negation measures or briefly , where a and b are symbols of any alphabet, will be called general or abstract, if a and b are exchanged by quantitative figure symbols, the measures will be called particular or concrete.

The whole variety of affirmation measures, expressed by quantitative symbols-figures such as a, forms a set of affirmation numbers D, a variety of the negation measures, expressed by symbols-figures such as bi, forms a set of negation numbers Ni. A set of affirmation-negation numbers or numerical opposites will be expressed by .

If the affirmation numbers describe the quantitative aspect of an object of thought, they will be called quantitative numbers, if they describe the aspect of the contents, they will be referred to contents numbers, in regard to the description of the material aspect, suitable names for affirmation numbers will be material numbers, etc. A set of negation numbers describes polar opposed sides and their names are polar contranly of those used for affirmation numbers. These are qualitative numbers, numbers of form, ideal numbers, etc

Additive q-times recurrence of any element a forms a quantitative additive degree of an judgement

, where . (1.57)

The basis a of the additive degree is the basis of the additive power and the additive exponent q is the recurrence superstructure. The additive power allows the judgement S to be expressed as the discrete quantitative sum:

(1.58)

with the expansion index q and the additive discreteness root

. (1.59)

Additive q-times recurrence of any element a is additive quantitative recurrence of the element a, which will be also called quantitative recurrence.

Along with the quantitative recurrence, we will introduce the concept of qualitative recurrence of the element a, ir-times qualitatively, according to the equality:

, where . (1.60)

Qualitative recurrence of an judgement are negation of quantitative recurrence Formula (1.60) allows realize qualitative discrete expansion of the judgement S:

(1.61)

with the expansion index ir and the additive discreteness root

. (1.62)

Combining quantitative and qualitative expansions, we obtain quantitative-qualitative expansions of the judgement S:

. (1.63)

with the discreteness index

(1.64)

and the additive discreteness root

(1.65)

Multiplicative q-times recurrence of the affirmation element a forms the multiplicative degree of the judgement

where . (1.66)

The basis of the multiplicative power is its base number and its superstructure is the exponent. Quantitative recurrences will be supplemented by polar-opposed qualitative recurrences

, where . (1 67)

Combining both recurrences, we have the quantitative-qualitative multiplicative recurrence

, where . (1.68)

Hence, we arrive at the multiplicative discrete expansions

(1.69)

with the multiplicative exponent and the discreteness root

. (1.70)

While making comparisons, quantitative multiplicative power oppositi-judgements with various affirmation bases should be reduced to a common reference basis e, chosen from specific considerations:

, (1.71)

where g is the index of expansion of the basis a in terms of the element e equal to logarithm of the basis a to the base e: .

, (1.72)

where is a differential of the variable unity: .

This limit, equal to the base of natural logarithms, is well-known. It simplifies a great many mathematical calculations and expressions, and it takes as a principle of the important physical laws and regularities.

A quantitative multiplicative power oppositus-judgement with the affirmation basis a, reduced to the reference basis e, is expanded into an infinite power series in terms of the power x:

(1.73)

Separating odd and even multiplicative discretenesses, we will express the series as the sum of two functions:

, (1.73a)

where

,

---------------------------------------------------------(1.74)

.

These variable opposites will be termed a hyperbolic cosine and a sine of X, respectively, in terms of the bases a. The relation between them

will be referred to as a hyperbolic tangent of a in terms of the base a.

The series (1.73), in which the expansion power is qualitative, will be taken as a measure of a qualitative multiplicative recurrence of the affirmation judgement:

(1.75)

Quantitative and qualitative components of the sum (1.75) define trigonometric cosine and sine of x in terms of the base a:

,

(1.76)

.

Thus, we have

(1.77)

or . (1.77a)

The judgement of the qualitative recurrence a is characterized by the ideal period

with the norm . (1.78)

The graph of such dialectical judgement S is shown in Fig. 1.7. If the space-time affirmation-negation judgement is projected onto the plane si-ino, we will have an infinite sheeted surface of events, every sheet of which expresses a moment. Since many events be repeated, the infinite-sheeted surface of events can be replaced by a factor-ized multisheeted surface of projections for simplified graphic comparison of contradictories elements of the opposites.

Multiplicative relations express a nonseparable bond, indivisibility, and systemability and can be used for creating quantitative-qualitative power judgements describing various contradictory processes and states of nature.

We will consider an elementary opposite of a nonrecurring-recurring process, for example, nonrecurring on the quantitative side and recurring on the qualitative side:

. (1.79)

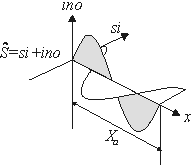
Fig. 1.7. A graph of a dialectical judgement.

The periodic semi-oppositus describes logic of the periodic aspect of the process with the ideal period

, where , (1.80)

on its qualitative side, while the semi-opposite expresses the nonrecurring facet of the process on the quantitative side. However, the nonrecurring facet of the process recurs quantitatively with the scale factor d:

,where and , (1.81)

and the additive material period Ò on the superstructure level:

. (1.82)

Theoretical Dialectical Journal: Physics-Mathematics-Logic-Philosophy, N.1, site http://www.tedial.narod.ru/